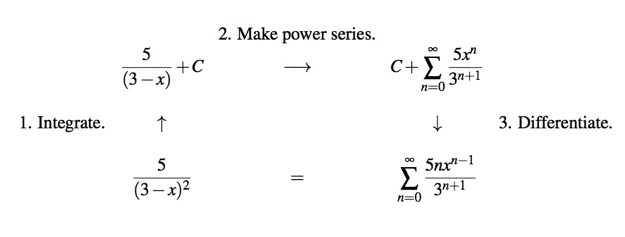Using the ease of moving between geometric series and their sums, plus the fact that for power series the derivative of a series is the sum of the derivatives of each term (and likewise for integrals), we can find a wider range of power series without too much trouble. I like making diagrams so here we go.

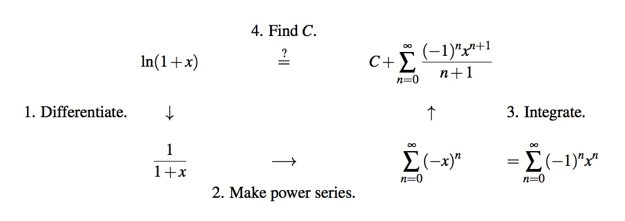
1. Find a power series representation of

This function differentiates to a fraction we can interpret as the sum of a geometric series.

To complete Step 4, note that for x=0, = 0 and the series also equals 0, so C must be 0. The radius of convergence for the series obtained in Step 2 is 1, with an interval of (-1, 1). When you integrate the interval of convergence can gain or lose endpoints, but that’s a small thing to check after this fairly quick conversion.

2. Find a power series representation of

Instead of differentiating, this time we integrate to get the sum of a geometric series.

In Step 3 note that from the perspective of the derivative operator is constant. There’s no constant of integration to find so once you’ve rounded the bases you’re done – well, except for thinking about the radius and interval of convergence.

One more after the jump.

3. Find a power series representation for

This time we differentiate twice.

Once again you do not have to find C at the end, but in this case it is because C stays undefined: the series is intended to equal an indefinite integral, not a specific function. C does not affect the radius or interval of convergence of the series.

We can use the series above to find a power series for the definite integral Since plugging 0 into the series obtained in Step 6 gives 0, the answer is the result of plugging in 1: